Geometry is a trending topic in Bank exams. In the series of sharing Geometry basics, today I am sharing rules related to Circles :-

Area of the circle is A=πr2
Circumference (perimeter) of the circle= 2πr
Sector of a Circle

To find the area of the sector we must know the central angle .Suppose the central angle is θ.

Chords of a Circle
The longest chord in a circle is diameter.
Based on the same logic , there can be many questions like :
Q1.
The two parallel chord of a circle are 10 and 12 cm and the radius of the circle is 13 cm. Find the distance between two chords .Solution : In this question there can be two possibilities about the locations of the chords .
- Both the chords are on the opposite side of the center.
- Both the chords are on the same side of the center.

Since OP is a perpendicular on the chord AB
Therefore AP=PB =12
In triangle OPB by Pythagorean Theorem
OP2 = OB2 –PB2
OP2 = OB2 –PB2
= 132-122
= 169-144
= 25
OP= 5 cm
Therefore CR=RD =5 cm
In triangle ORD by Pythagorean theorem
OR2 = OD2-RD2
= 132-52
= 169-25
=144
OR = 12
Therefore the distance between the chords AB and CD = OP+OR =5+12=17 cm
Now let us do it with second possibility
In this case also OP and OR will remain 5 cm and 12 cm respectively.
We need to find out the PR
PR = OR –OP = 12-5 = 7 cm
Therefore the distance between the two chords = 7 cm
Angles in a circle
Angles in the major segement
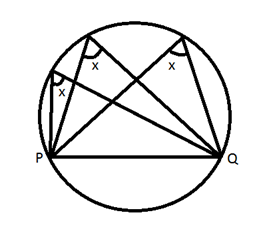
RULE 1:
According rule the angles made in the same segments are equal.
OR
Angles made by the same chord in the same segment are always equal.
Remember that there can be any number of angles but if they are
in the same segment, they are always equal.
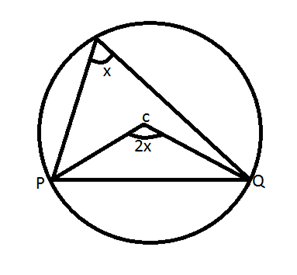
RULE 2:
Angles made by the chord at center are twice the angle made at the circumference.
Angles in the minor segment