1.
A person travels from A to B at a speed of x km./h and returns B to A at a speed of y km./h, then his average speed:

Example-
If Reeta travels from Goa to Delhi at a speed of 105 km./h and from Delhi to Goa at a speed of 120 km./h. Find his average speed.
Solution:
Solution:
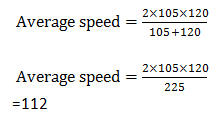
2.
If a person travels three equal distance at a speed of X km./h, Y km/h and Z km/h respectively, then average speed:

Example.

Example.
If Mahima travels three equal distance at a speed of 120 km/h, 125 km/h and 140 km/h respectively. Find her Average speed.
Solution:
Solution:

3.
If the distance ‘A’ is covered with a speed of x km/h, distance ‘B’ is covered with a speed of y km/h and distance ‘C’ is covered with a speed of z km/h, then average speed:

Example-

Example-
If the distance 75 km is covered with a speed of 25 km/h, distance 40 km is covered with a speed of 20 km/h and distance 60 is covered with a speed of 20 km/h. Find the average speed.
Solution:
Solution:
The average of a items is x and the average of b item is y, then total average of (a+b) is

Example-

Example-
If the average salary of 7 month is 25200 and the average salary of 5 month is 24000. Find the average salary of 12 months.
Solution:
Solution:

5.
There are total ‘x’ members and their total average age is ‘a’ years. Then a new member joins and the new average age of the members become ‘n’, then the age of new member that joins will be
= [n+x(n-a)] years
Example-
= [n+x(n-a)] years
Example-
If the average age of 15 students in a class is 20 and a teacher joins the class then their average age increase by 1. What will be the age of teacher?
Solution:
After joining the teacher new average= 20+1 = 21 years
The age of teacher = 21+15(21-20)
=21+15
=36 years
Solution:
After joining the teacher new average= 20+1 = 21 years
The age of teacher = 21+15(21-20)
=21+15
=36 years
6.
There are total ‘x’ members and their total average age is ‘a’ years. Then a member leaves and the new average age of the members become ‘n’, then the age of new member that joins will be
= [n-x(n-a)] years
Example
= [n-x(n-a)] years
Example
A class of 19 children and a teacher, the average weight is 48 kg. If the weight of the teacher is excluded, then the new average weight becomes 48.5. What is the weight of the teacher?
Solution:
=48.5-20(48.5-48)
=48.5-10
=38.5 years
Solution:
=48.5-20(48.5-48)
=48.5-10
=38.5 years
Some important points:
7.
If the value of each item is increased by the same value, then their total average will also be increased by that same value.
Example
Example
The Average of five numbers 55,45,65,95,85 is 69. If each number is increased by 5, then what is the new average?
Solution:
New average= 69+5= 74
Solution:
New average= 69+5= 74
8.
If the value of each item is decreased by the same value, then their total average will also be decreased by that same value.
Example-
Example-
The Average of five numbers 55,45,65,95,85 is 69. If each number is decreased by 5, then what is the new average?
Solution:
New average= 69-5= 64
Solution:
New average= 69-5= 64
9.
If the value of each item is multiplied by the same value, then their total average will also be multiplied by that same value.
Example-
Example-
The Average of five numbers 55,45,65,95,85 is 69. If 5 is multiplied with each number then what is the new average?
Solution:
New average= 69×5= 345
Solution:
New average= 69×5= 345
10.
If the value of each item is divided by the same value, then their total average will also be divided by that same value.
Example-
Example-
The Average of five numbers 55,45,65,95,85 is 69. If 5 is divided from each number then what is the new average?
Solution:
New average= 69/5= 13.8
Solution:
New average= 69/5= 13.8




